Recently, scientists have discovered a strange phase of matters, in which electric current flows in one spatial direction like it would in metals and the other as insulations [1]. In a recent PRL paper [2], Zhao et. al. have used an advanced mathematical method to explain this mysterious electric conduction phenomenon, that may have potential applications for future quantum devices. They have studied a quantum system composed of two sublattices, where sites in one lattice only couple to sites in the other [3]. The electric conduction of such quantum systems is entirely governed by a vortex and its spatial proliferation, which is commonly seen as natural occurrence such as whirlpools in oceans. The authors discovered that due to a topological quantum interference effect, pairs of vortices can become spatially polarized, and the proliferation of the polarized vortex pairs yields the strange electric conduction phenomenon. Their findings have wider implications for a range of physical phenomena, encompassing the electric conduction in quantum systems as well as wave phenomena in open classical systems, where energy may dissipate as a result of interactions with the surrounding environment.
Transport phenomena lie at the heart of physics, and transports of disordered topological systems have been extensively studied for last decades. Nonetheless, the role of the band topology in the Anderson transition has been elusive, and it has been widely believed that the localization-delocalization transition of a wave function occurs simultaneously in all the spatial directions. Contrary to this conventional wisdom, Xiao et.al. found in 2023 that the metal-insulator transition becomes two-step transitions in the presence of the 1D weak band topology, where a new thermodynamic phase, dubbed as “quasi-localized” phase, universally emerges between metal and Anderson localized phases in phase diagrams [1] (see Fig. 1). In the quasi-localized phase, an exponential localization length along a spatial direction with the 1D weak topology (dubbed as “topological direction”) is divergent, while the localization length along the other directions is finite. Xiao et. al. also clarified that a transition between metal and quasi-localized phases belongs to a different universality class from the Anderson transition of the same chiral symmetry class without the weak topology. These numerical discoveries clearly demonstrate that the 1D weak topology alters the nature of the Anderson transition in the chiral symmetry classes completely, yielding even the new phase between metal and insulator.
In the recent PRL paper [2], Zhao et. al. explained the universal emergence of the quasi-localization phenomenon from a lens of a topological field theory. Inspired by a theory showing that the Anderson transition in 2D chiral symmetry classes is instigated by spatial proliferation of vortex excitations associated with a U(1) subgroup symmetry in a non-linear sigma model [4], Zhao et. al. pointed out that the 1D weak topology confers a quantal (``Berry'') phase upon a vortex-antivortex pair, dependent on its spatial polarization relative to the topological direction. They argued that the quantal phase results in destructive interference among configurations of those pairs that are not parallel to the topological direction, effectively polarizing the pairs along the topological direction (see Fig. 2). The proliferation of the polarized vortex-antivortex pairs naturally renders correlations of a field variable to be weakly and strongly disordered along the topological direction and the other direction(s), respectively. Consequently, the quasi-localized phase with the extremely anisotropic electric conduction universally emerges next to the metal phase in a global phase diagram for chiral symmetric models with the 1D weak topology. Motivated by this argument, Zhao et. al. formulated a set of renormalization group (RG) equations for the 2D non-linear sigma model in chiral unitary class with 1D weak topology, and confirmed that the quasi-localized phase always appears next to the metal phase in a RG phase diagram (see Fig. 3).
Thanks to an article by Luo et. al. [5], it is also expected that the quasi-localization phenomena in the chiral symmetry classes can be ubiquitously realized in eigenstates of non-Hermitian operators in the most fundamental symmetry classes, such as classes A, AI, and AII. This indicates a broader applicability of the theory to wave phenomena in open classical wave systems as well as open quantum systems, where the energy dissipates due to their coupling with the thermal bath.
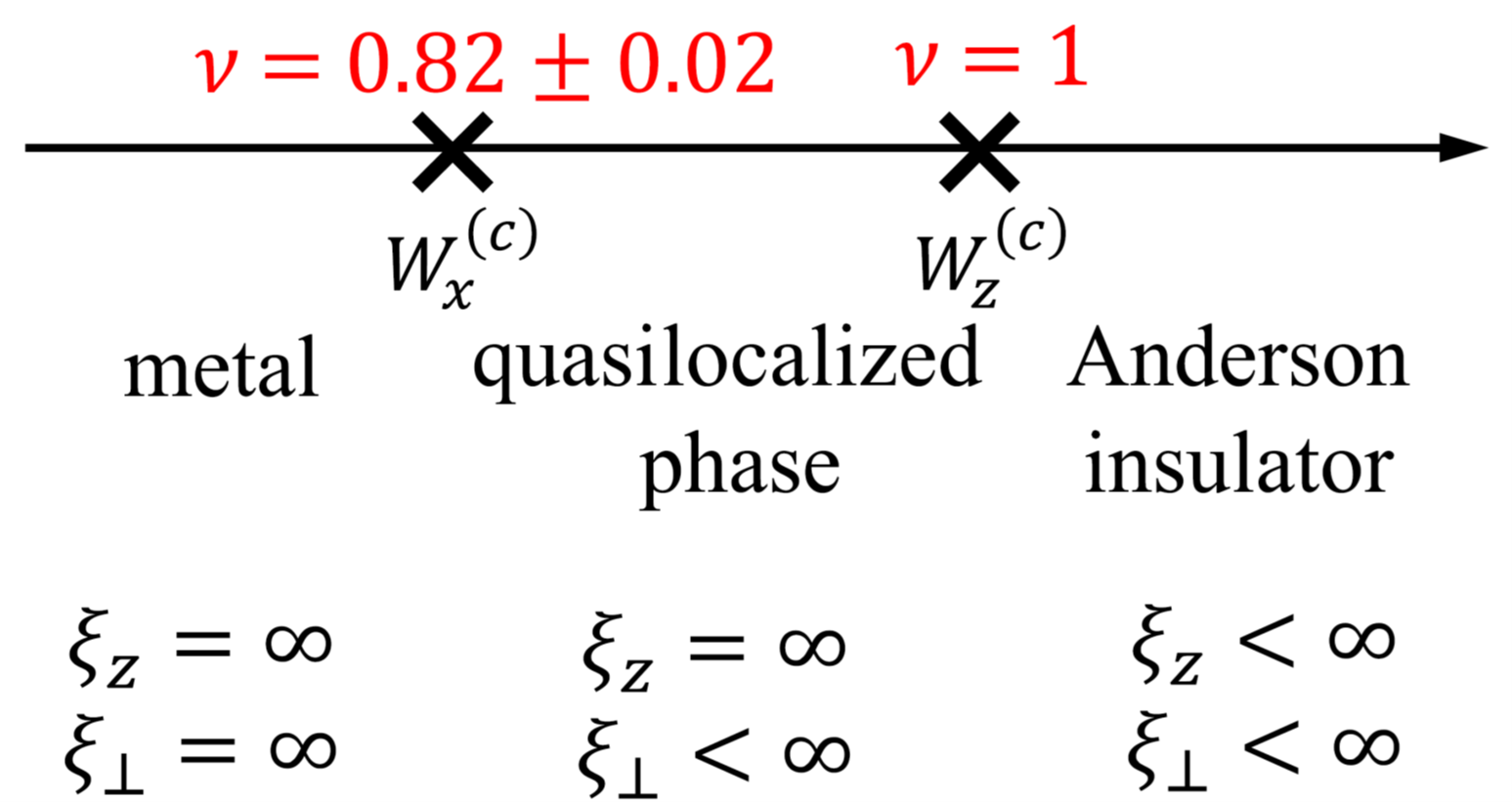
Fig. 1. A schematic phase diagram of chiral symmetric systems with 1D weak topology. The quasi-localized phase with divergent localization length xz along the topological direction, and finite localization length x^ along the other directions universally emerges between metal and localized phase. n =0.82 is an estimated critical exponent of a transition between metal to quasi-localized phases in the three dimension, while n=1 is an exact critical exponent of a transition between quasi-localized and localized phases.
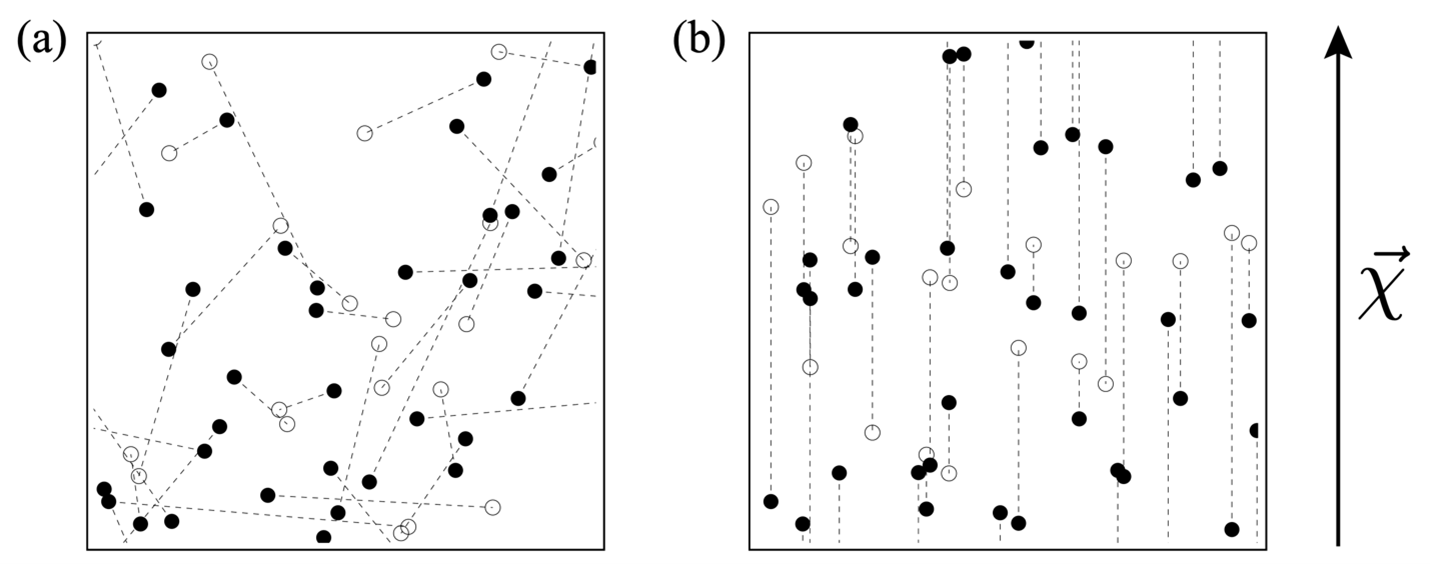
Fig. 2. Schematic pictures show spatial proliferations of vortex-antivortex pairs in chiral symmetric systems without (a) and with (b) the 1D weak topology. Black filled circle and white blank circle connected by a dotted line is a pair of vortex and antivortex. A vector c specifies the topological direction with the 1D weak topology.
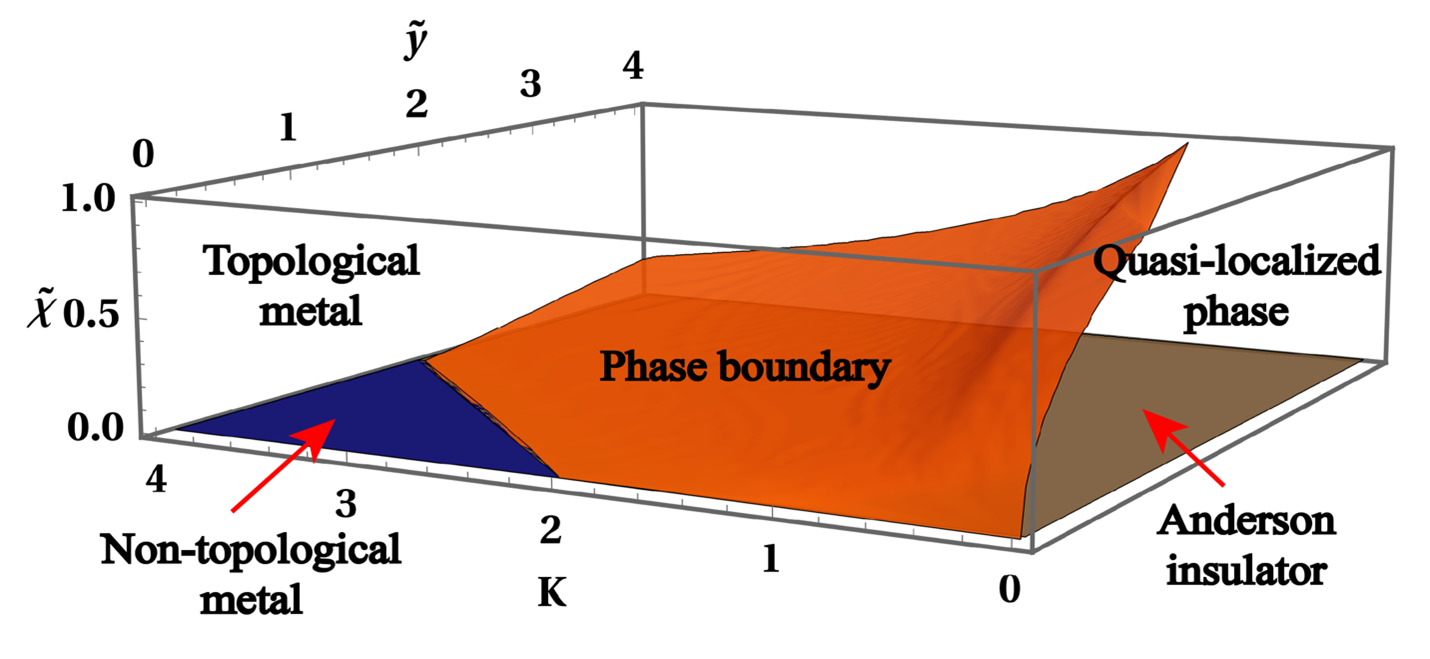
Fig. 3. Phase diagram of two-dimensional disordered models in chiral unitary class obtained from the renormalization group equations. c is the 1D weak topology strength, y is a fugacity that controls the proliferation of vortices, and a stiffness K describes the stability of conductivity against the vortices.
The paper is published in Phys. Rev. Lett. …., where Pengwei Zhao [2021 ICQM graduate student] is the first author of this article. Zhenyu Xiao and Yeyang Zhang [2020 ICQM graduate students] are co-authors, while Dr. Ryuichi Shindou [associate professor in ICQM, School of Physics] is a corresponding author of the article. This project is supported by the National Basic Research Programs of China [No. 2019YFA0308401] and by the National Natural Science Foundation of China.
References:
[1] “Anisotropic Topological Anderson Transitions in Chiral Symmetry Classes”, Z. Xiao, K. Kawabata, X. Luo, T. Ohtsuki, and R. Shindou, Phys. Rev. Lett. 131, 056301 (2023).
[2] “Topological effect on Anderson Transition in Chiral Symmetry Classes”, P. Zhao, Z. Xiao, Y. Zhang, and R. Shindou, Phys. Rev. Lett. 133, 226601 (2024).
[3] Researchers call such quantum systems as “systems in chiral symmetry classes” or “chiral symmetric systems”. So shall we do in the rest of this article.
[4] “Metal-insulator transition in two-dimensional random fermion systems of chiral symmetry classes”, E. J. Koenig, P. M. Ostrovsky, I. V. Protopopov, and A. D. Mirlin,
Phys. Rev. B 85, 195130 (2012).
[5] “Unifying the Anderson transitions in Hermitian and non-Hermitian systems”, X. Luo, Z. Xiao, K. Kawabata, T. Ohtsuki, and R. Shindou, Phys. Rev. Research 4, L022035 (2022).
