The quasiparticles in two-dimensions can obey anyon statistics due to the topology of its moving trajectory. The anyon statistics in two spatial dimensions is distinctly different from both the Bose-Einstein statistics and the Fermi-Dirac statistics. The anyon could be classified into Abelian anyon and non-Abelian anyon, where the swapping (braiding) operation of the non-Abelian anyons’ spatial positions will lead to a non-trivial wave function evolution and give rise to a new quantum state. Based on such peculiar properties, one can encode information in the non-Abelian anyons and the corresponding manipulation (calculation) could be proceeded via the braiding operations. Such method of information storage and manipulation, known as “topological quantum computation”, possesses great advantages including fault tolerance owing to the protection of the non-trivial topology. Therefore, topological quantum computation has becoming an important field in the research of quantum computation.
In the last two decades, the researches on topological quantum computation mainly focused on the Majorana zero-mode (or chiral Majorana edge mode). Majorana fermion’s antiparticle is itself, and the realization of Majorana fermion in condensed matter system strongly relies on the presence of superconducting order parameter. Recently, Prof. Xin-Cheng Xie from Peking University and his collaborators put forward a novel idea realizing non-Abelian braiding in Dirac fermionic modes, which are elementary excitation modes in non-Majorana system (non-superconductivity system). This work has been published in Physical Review Letters [Phys. Rev. Lett.125, 036801.https://link.aps.org/doi/10.1103/PhysRevLett.125.036801].
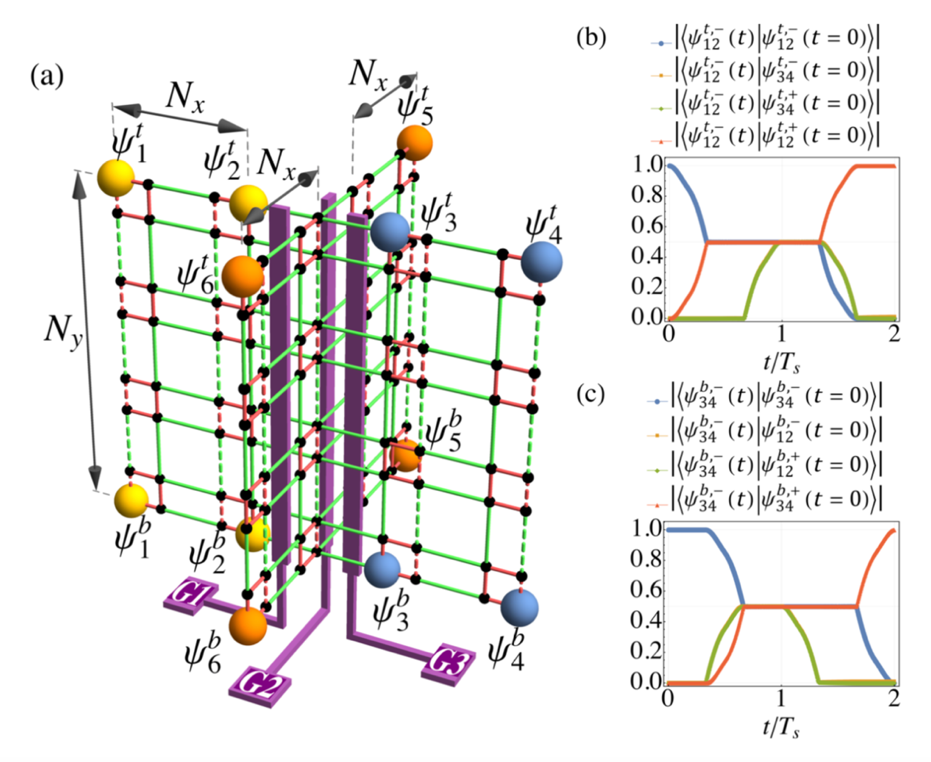
(a) Schematic plot of higher-order topological insulator nanostructure supporting non-Abelian braiding;
(b), (c) The non-trivial wave function evolution during the non-Abelian braiding process.
The incarnation of Dirac fermionic modes in condensed matter physics could be the topologically protected zero-energy boundary states in topological insulators. For instance, the topological corner states in higher-order topological insulator, which has been drawing extensive attention in recent years, is a kind of typical Dirac fermionic mode. By numerically simulating the evolution of the topological corner states’ wave function during the braiding, Prof. Xin-Cheng Xie and his collaborators have demonstrated the non-Abelian braiding properties of the topological corner states. In addition to the numerical calculations, this work has also analytically investigated the Dirac fermionic modes bounded with the half-flux vortex in two-dimensional topological insulators. Such vortex-bounded Dirac fermionic modes are highly similar to the topological corner states for both their topological origin and mathematic forms. In the assistance of these vortex-bounded Dirac fermionic modes, not only the Dirac fermionic modes’ non-Abelian statistics but also the close relation between the non-Abelian statistics and the topologically-induced geometric phase is revealed in an analytical fashion.
Based on these results, the authors claimed that in the non-Majorana system (non-superconductivity system), topologically protected Dirac fermionic modes also support non-Abelian braiding properties. Compared with the Majorana zero-modes, the Fock space constructed by the Dirac fermionic modes is in the different quantum dimensions. The explicit form for both the braiding operator and the braiding matrix of the Dirac fermionic modes are also different from the Majorana ones. Experimentally, the Dirac-fermionic-mode-based non-Abelian braiding possesses several advantages such as superconductivity-free, larger energy gap, and shorter braiding time-cost. One-dimensional Su-Schrieffer-Heeger model and higher-order topological insulator can be promising experimental platforms realizing the non-Abelian braiding of Dirac fermionic modes. In conclusion, this work opens up a new avenue realizing non-Abelian braiding and topological quantum computation.
Yijia Wu (formerly Ph. D. candidate, now postdoctor in Prof. X. C. Xie’s group) is the first author, and Prof. X. C. Xie is the corresponding author of this Letter. Other collaborators including Prof. Hua Jiang (Soochow University), Prof. Jie Liu (Xi’an Jiaotong University), and Prof. Haiwen Liu (Beijing Normal University). This work is financially supported by the National Natural Science Foundation of China.
